Hog Health
thumbnail
Livestock Management
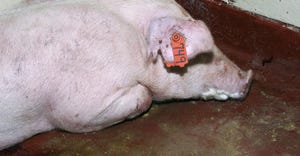
Livestock producers find partner in ISU Veterinary Diagnostic LaboratoryLivestock producers find partner in ISU Veterinary Diagnostic Laboratory
More than 90% of the lab’s cases come from animals being raised for food, and about 75% of the overall cases are swine.
Subscribe to Our Newsletters
National Hog Farmer is the source for hog production, management and market news









.jpg?width=300&auto=webp&quality=80&disable=upscale)